

 Very large floating structures (VLFS) are being considered as a new airport, storage and manufacturing facilities, and so on. Studies on their safety and performance in various circumstances are of primary importance in the design. The configuration of VLFS considered here is of pontoon type, and its typical size will be 5 km long, 1 km wide, and only a few meters deep. Therefore this type of structure is featured in that:
Very large floating structures (VLFS) are being considered as a new airport, storage and manufacturing facilities, and so on. Studies on their safety and performance in various circumstances are of primary importance in the design. The configuration of VLFS considered here is of pontoon type, and its typical size will be 5 km long, 1 km wide, and only a few meters deep. Therefore this type of structure is featured in that:
 The equation of elastic motion is solved by adopting the mode-expansion method. The modal functions used are the products of so-called free-free beam modes and the free-end conditions along the edge are satisfied as natural boundary conditions in transforming the stiffness matrix by partial integrations.
The equation of elastic motion is solved by adopting the mode-expansion method. The modal functions used are the products of so-called free-free beam modes and the free-end conditions along the edge are satisfied as natural boundary conditions in transforming the stiffness matrix by partial integrations.
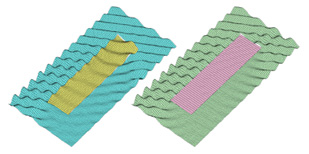
Shown on the left of two figures is the elastic deflection of a structure of L/B=4 (yellow part) and the wave elevation around the structure (blue part) in a plane progressive wave of L/lambda=10 and incidence angle of 30 degrees.
For a comparison, the corresponding wave pattern around a rigid plate is shown on the right of the figures.
We can see that the wave reflection from the bow part is small and a part of the wave propagates beneath a flexible plate. Furthermore, the wavelength of the elastic wave on a structure is longer than that of the incident wave and the propagation angle bends to the longitudinal direction of the structure.
Furthermore, by computing the memory-effect function in terms of the Fourier transform of computed results in the frequency domain and by solving the motion equations containing convolution integrals associated with memory effects, numerical simulations can be implemented for transient elastic responses of a floating airport due to an impulsive or arbitrary moving load. For example, some simulation results for the take-off of Boeing 747 jumbo jet are shown below. (To make the vertical deflection visible, the scale for the elastic deflection is amplified 5,000 times. Thus the actual deflection in the vertical direction is very small actually.)

 Link to
related References
Link to
related References
 Home Page of Professor Kashiwagi
Home Page of Professor Kashiwagi
